
The Sky of Math Is Full of Stars
 |
| Erio Castagnoli |
There are people who are able to find beauty in the rigorous and elegant formalism of mathematics, just as others find beauty in nature or art (some even argue that math is art, or nature, or both). The dearly missed Bocconi Professor Erio Castagnoli was surely a math lover, and was able to transmit his love and passion for mathematics to several generations of Bocconi students. Many of these have become leading scientists and faculty members in top universities all over the world. Castagnoli's research activity has touched on many important disciplines, ranging from decision sciences to mathematical finance. However, the common trait that characterized his approach is a taste for mathematical abstraction, that is the art of identifying a unifying scheme to frame a multiplicity of phenomena.
Even though he left us in 2019, Castagnoli's ideas continue to live on and yield important scientific developments. A recent paper co-authored by Giacomo Cattelan (New York University), Fabio Maccheroni (Bocconi), Claudio Tebaldi (Bocconi) and Ruodu Wang (University of Waterloo, Canada) is based on an intuition that came to him in the last days of his life. It investigates star-shaped measures and illustrates their application to finance and decision theory.
To get an idea of a star-shaped measure, consider an illiquid market. If you want to double the purchased quantity of a given good, then you must expect to pay more than twice the original price. In fact, as the purchased quantity increases, the good in question gets scarcer and consequently its unit price also increases. The mathematical translation of this phenomenon is that the price-quantity relationship is convex. However, the abovementioned economic mechanism does not require that convexity is attained everywhere. If the price-quantity function is normalized, i.e. set to zero at zero, then convexity is required only at zero. Functions with this latter property are called star-shaped at the origin. Interestingly, all normalized convex functions are star-shaped at the origin, but the reverse does not hold in general.
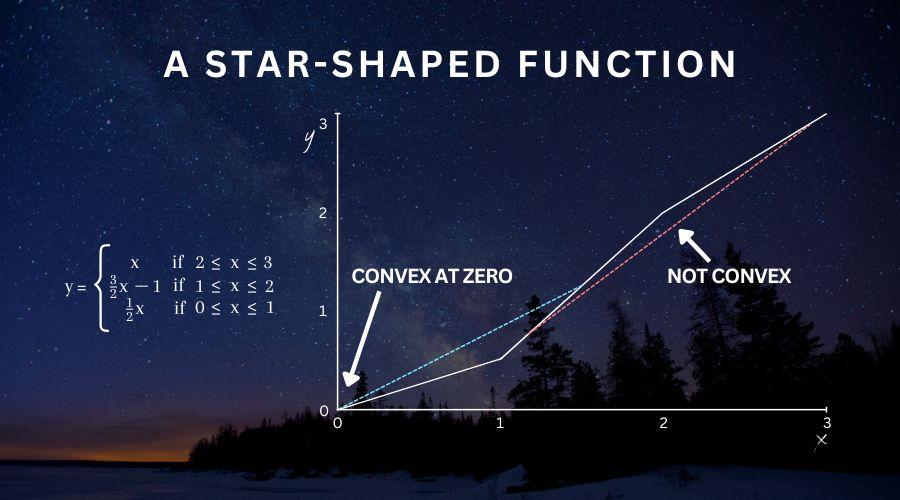
One may wonder whether star-shaped but non-convex functions are relevant for applications. A good starting point to understand their importance is the fact that any realistic risk measure is star-shaped. Specifically, to prevent the lack of liquidity during a default from propagating the crisis to the financial system, current regulation requires central counterparty clearing houses to deposit a certain amount of money as collateral (so-called margin requirements). In fact, it can be shown that these requirements must be the result of a star-shaped, but not necessarily convex, function with respect to the size of the trades.
Moreover, the appeal of star-shaped risk measures also has a decision-theoretic foundation. They describe institutional decision makers who, in the face of uncertainty, will take any available measure to mitigate its adverse effects. As the total capital of the financial institution increases, the decision makers will decrease the fraction of capital that is exposed to those effects.
"The main message of the paper," explain Tebaldi and Maccheroni, "is that less is more: the weaker requirement of star-shapedness is often sufficient to model many interesting examples of violations of convexity that are relevant for economic practice. This last work that we co-authored with Professor Castagnoli highlights once again his enduring inheritance: the beauty of mathematics is key to knowledge that matters."
Erio Castagnoli, Giacomo Cattelan, Fabio Maccheroni, Claudio Tebaldi, Ruodu Wang (2022). "Star-Shaped Risk Measures." Operations Research 70(5): 2637-2654. https://doi.org/10.1287/opre.2022.2303.